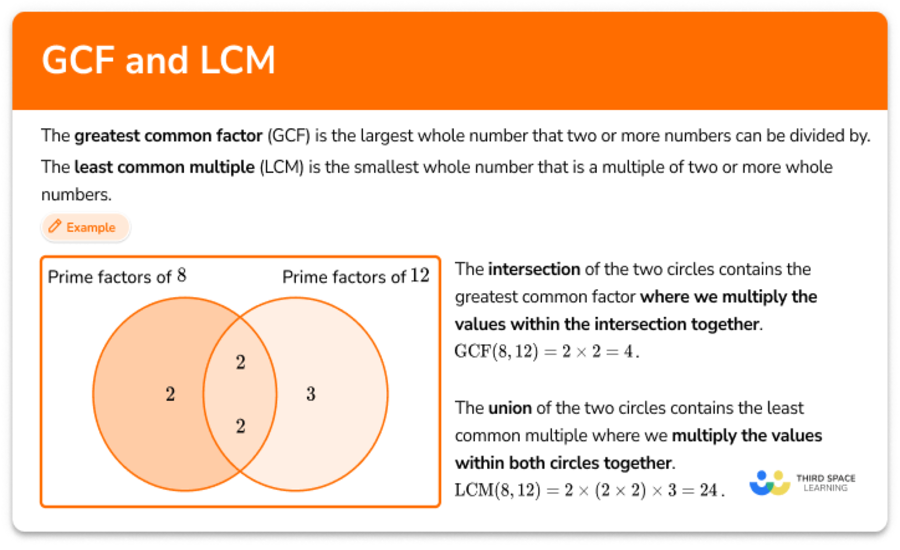
The Difference Between GCF and LCM: Understanding Key Math Terms
Mathematics is a complicated subject. Understanding some mathematical terms, the concepts behind them, and the formulas that can be used to calculate them can be tricky for many people. Many consider such terms abstract concepts and don’t pay much attention to them when studying mathematics.
However, when we step into professional life, knowing the usefulness of those apparently abstract terms makes us regret overlooking them during studies. GCF and LCM are also amongst those unnoticed key math terms. If you are one of those who didn’t pay much attention to concepts like GCF and LCM, this article is here to help you.
It will not only discuss GCF and LCM but also highlight the difference between these key math terms. So, make sure you stick to it to understand these essential concepts and methods of calculation. Further details are given below.
GCF (Greatest Common Factor)
Also known as HCF, which stands for ‘Highest Common Factor’. It is a primary mathematics term used to denote the largest number one can use to divide multiple non-zero integers evenly. Mind you, a number will only be termed a GCF or HCF if it divides all the given non-zero integers without a remainder. The GCF concept is considered one of the key principles in mathematical calculations involving integers. In simple terms, you have to identify the greatest variable amongst the common variables capable of evenly dividing the given numbers.
Calculating GCF
You can use multiple methods to manually calculate GCF or HCF of the given numbers. We will discuss the frequently used ones in detail. Read on to learn more.
Listing Factors: The first method for finding the GCF of two or more non-zero integers involves listing their factors or devisors. For instance, we have to find the GCF of 12, 30, and 42.
Factors of 12 are 1, 2, 3, 4, 6, 12.
Factors of 30 are 1, 2, 3, 5, 6, 10, 15, 30.
Factors of 42 are 1, 2, 3, 6, 7, 14, 21, 42.
Once you identify the factors of all numbers, it is time to filter common devisers of all the given integers.
The common factors are 1, 2, 3, and 6. As the greatest among common devisers is 6, it is also the GCF of 12, 30, and 42.
Prime Factorization Method: The prime factor decomposition method is another common method for manually calculating the GCF of given numbers. This method involves the decomposition of given integers into prime factors. According to basic mathematical concepts, any positive non-zero number could be a prime number or a product of multiple prime integers. So, the prime factor decomposition method allows us to easily find the HCF of given numbers. Here is how you can do it.
We have to find the GCF of 12, 18, and 24. We will decompose numbers into prime factors to get GCF.
12 = 2 x 2 x 3
18 = 2 x 3 x 3
24 = 2 x 2 x 2 x 3
The common prime factors in decomposing all three integers are 2 and 3. We have to calculate the highest power of both these prime factors in the decomposition of all three numbers. So the highest power of both these numbers is 1. Hence, the product of the highest common power of prime factors in the decomposition of given integers will be the GCF, which is 2 x 3 = 6.
Easier Calculation of GCF
Considering the above calculations, it is pretty evident that manually calculating the GCF of larger integers, such as 3 or 4-figure numbers or even larger ones, could be tricky. Chances of mistakes also exist. Hence, automated calculation is the way to go. We don’t suggest using offline calculators for this purpose, as they also make the process laborious and time-consuming. A simple and swift way to calculate the GCF of larger numbers is using https://greatestcommonfactorcalculator.info/. Here are some benefits of using it.
- Quick output.
- Accurate calculations.
- Easy accessibility through various devices.
- Ease of use.
LCM (Least Common Multiple)
Like GCF, LCM is also a fundamental mathematical concept many of us considered abstract during our primary studies. Some people often end up confusing both of these terms, but the concept of LCM is far different from that of HCF. LCM, or least common multiple, is the smallest number among the given integers’ common multiples. The LCM of given numbers could be equal to one or larger than all given integers. It is worth mentioning that a multiple is a number obtained due to the multiplication of a number with itself or another.
Methods to Find LCM
Multiple methods are available to help you calculate the least common multiple of given numbers, including listing multiples, prime factor decomposition, and division methods. We will only demonstrate LCM calculation using the listing multiples and prime factorization method. So here they are.
Listing Multiples Method: Just like the listing factors or devisers method we use to calculate GCF, calculating LCM requires us to list multiples of various numbers until we get the smallest of the common multiples of the given numbers. Here is how you can do it for 12, 24, and 36.
Multiple of 12 are 12, 24, 36, 48, 60, 72, 84, 96
Multiples of 24 are 24, 48, 72, 96
Multiples of 36 are 36, 72, 108
The common multiple of all the given numbers is 72, and it is also the smallest common multiple, so it is the LCM of 12, 24, and 36.
Prime Factorization Method: The Prime factorization method works quite similarly to the one we use to calculate GCF. The only difference is that we take the highest power of the prime factor appearing in the decomposition of any of the given numbers. So here it is
12 = 2 x 2 x 3
24 = 2 x 2 x 2 x 3
36 = 2 x 2 x 3 x 3
Considering the above calculations, the highest powers of 2 and 3 appearing in the above calculations as 3 and 2, respectively. So, the LCM of 12, 24, and 36 would be the product of 23 and 32, which is 72.
Accurate LCM Calculation
A better way to calculate the LCM of a larger number is by leveraging automated calculation. Like the online greatest common factor calculator, you can also use a web-based least common multiple calculator. It will help you ensure accurate results. The benefits of using this online tool are similar to those of the GCF calculator.
Conclusion
Considering concepts like GCF and LCM abstract could be a false approach. These concepts find various applications in practical life. Hence, you must know the nitty-gritty of HCF and LCM and their differences. You must also learn different methods to calculate them. We have tried to help you recall these concepts and understand their calculation methods by comprehensively discussing them in this article. Hopefully, you will find it helpful!